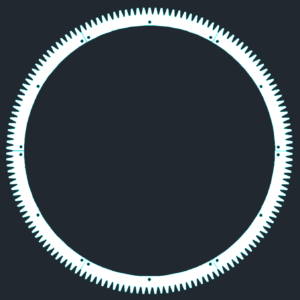
A worm gear on the rotor’s shaft engages with a spur gear like this on the rightmost digit wheel. The number of teeth on the spur gear is the same as the number of rotor revolutions needed for exactly one rotation of the digit wheel.
The rotor on top of Miles spins a worm gear. I want that worm gear to rotate the rightmost digit wheel once for each mile of air that passes by. To get that desired rotation, I need to mount an ordinary gear on that digit wheel. How many teeth must that gear have? Here’s how I approximated that number.
A mile of air is 5,280 feet long.
MileOfAir = 5,280 feet
The radius of the rotor is 3 feet, making its diameter 6 feet. Then the path followed by a rotor cup during one revolution of the rotor is 6π ≈ 18.85 feet.
Circumference ≈ 18.85 feet
One would think that the rotor will revolve once whenever 18.85 feet of air pass by, but the rotor is not entirely efficient. Assume that the rotor is 50% efficient.
EfficiencyOfRotor = 50%
That means that it only rotates halfway for every 18.85 feet of air. So for a full revolution, twice as much air must pass by. To put this relationship in an equation, one divides the Circumference by the Efficiency of the rotor.
AirPerRevolution = Circumference / EfficiencyOfRotor
which gives us
AirPerRevolution = 18.85 / 50% = 37.7 feet
So the rotor revolves once after the passage of 37.7 feet of air, and a mile of air has 5,280 feet. I can get the number of times that the rotor revolves during the passage of one mile of air by dividing 5,280 by 37.7. The result also is the number of teeth needed in the gear that engages the rotor’s worm gear.
NumberOfTeeth = MileOfAir / AirPerRevolution = 5,280 / 37.7 ≈ 140
That quantity uses my assumption that the rotor’s efficiency is exactly 50%, but I don’t know the efficiency precisely. So I’ll build the rotor first, mount it on my pickup truck, and have a passenger count the number of revolutions that it makes for each mile driven.

The spur gear can be constructed from six pieces to avoid wasting material. If the total number of teeth needed is a multiple of six, then all pieces are identical.
To save material, I’m going to fabricate the gear in six pieces. The image of the gear at the top of this page has 150 teeth because I don’t yet know how many teeth are needed, and it’s easier to draw a gear from six identical pieces. If necessary, I’ll create six different pieces, but for now I’ll hope that the driving test of the rotor yields a number of teeth that is divisible by six.